- Home
- Constraints
- Fit Results
- Historical Plots
- Papers and Conferences
- CKM Formalism
- Statistical Method
- Contacts
- Feedback
Gamma Combination from the UTfit goup
We use the relevant post-Moriond 2012 HFAG averages as inputs.
The results of combination:
| Parameter | Full fit |
|---|---|
![\gamma [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_d441395f3c6b5d6d14b98a13ae4b8837.png) |
 |
![\delta_{B}(DK) [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_41e661b01cefdd9ec2084738b64160f3.png) |
 |
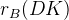 |
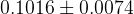 |
![\delta_{B}(DK^{*}) [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_1baa48393b03b96f22571066583a25b8.png) |
 |
 |
 |
![\delta_{B}(D^{*}K) [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_5211b61dcb830d9a98ee9a7878d74aff.png) |
 |
 |
 |
|
|
|
|
|
|
|
The angle  of the CKM triangle can be measured comparing
of the CKM triangle can be measured comparing  and
and  mediated transitions in
mediated transitions in  decays. The decays proceed through the following diagrams:
decays. The decays proceed through the following diagrams:

These diagrams are practically free from the New Physics contribution.
There are three methods to extract relevant information, each of them deals with its own  decay:
decay:
 and the relative
and the relative  conserving phase
conserving phase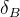 between the two amplitudes. These parameters depend on the
between the two amplitudes. These parameters depend on the  decay under investigation.
decay under investigation.
 of the CKM triangle can be measured comparing
of the CKM triangle can be measured comparing  and
and  mediated transitions in
mediated transitions in  decays. The decays proceed through the following diagrams:
decays. The decays proceed through the following diagrams:

 decay:
decay:
- a singly Cabibbo-suppressed CP eigenstate, like
 for Gronau-London-Wyler (GLW) method;
for Gronau-London-Wyler (GLW) method;
- a doubly Cabibbo-suppressed flavor eigenstate, like
 for Atwood-Dunietz-Soni (ADS) method;
for Atwood-Dunietz-Soni (ADS) method;
- a Cabibbo-allowed self-conjugate 3-body state, like
 for Giri-Grossman-Soffer-Zupan (GGSZ) method.
for Giri-Grossman-Soffer-Zupan (GGSZ) method.
 and the relative
and the relative  conserving phase
conserving phase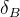 between the two amplitudes. These parameters depend on the
between the two amplitudes. These parameters depend on the  decay under investigation.
decay under investigation.
The Gronau-London-Wyler (GLW) method (M. Gronau, D. Wyler, Phys. Rev. Lett. B {\bf 253} (1991) 483; M. Gronau, D. London, Phys. Rev. Lett. B {\bf 265} (1991) 172) is based on the reconstruction of the  decay to
decay to  , where
, where  and
and  decay
to
decay
to  -even or
-even or  -odd eigenstates. The
-odd eigenstates. The  modes normally used are:
modes normally used are: 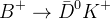 , with
, with  is also reconstructed.
The four observables for this method are formed in the following
way:
is also reconstructed.
The four observables for this method are formed in the following
way:
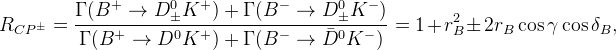
 This set can provide an information on
This set can provide an information on  ,
, 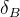 , and
, and  with an 8-fold ambiguity for the phases.
with an 8-fold ambiguity for the phases.
 decay to
decay to  , where
, where  and
and  decay
to
decay
to  -even or
-even or  -odd eigenstates. The
-odd eigenstates. The  modes normally used are:
modes normally used are: -
 :
:  ,
,  ;
;
-
 :
: 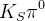 ,
,  ,
,  ,
, 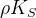 , and
, and 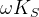 .
.
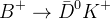 , with
, with  is also reconstructed.
The four observables for this method are formed in the following
way:
is also reconstructed.
The four observables for this method are formed in the following
way:
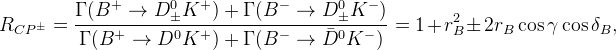
 This set can provide an information on
This set can provide an information on  ,
, 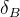 , and
, and  with an 8-fold ambiguity for the phases.
with an 8-fold ambiguity for the phases.
Error: File attachment at http://utfit.org/foswiki/pub/UTfit/repository/gamma_ggsz.txt, does not exist
Powered by
 Ideas, requests, problems regarding this web site? Send feedback
Ideas, requests, problems regarding this web site? Send feedback
 Ideas, requests, problems regarding this web site? Send feedback
Ideas, requests, problems regarding this web site? Send feedback

![\,\gamma [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_346b4369d40fd8964bda0f769a5b4bbe.png)

![\,\delta_{B}(DK) [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_de1251af0a072fb83f6dd951669769db.png)



![\,\delta_{B}(DK^{*}) [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_5164e268a514bb26250f2bad6b8038e2.png)



![\,\delta_{B}(D^{*}K) [^{\circ}]](/foswiki/pub/UTfit/GammaFromTrees/_MathModePlugin_59f1351d3c4dd771bf1d0e886a13457a.png)


